Antoine Chan-Lock, PhD

Computer scientist

Universidad Rey Juan Carlos
antoine.chanlock[at]gmail.com

Bio

I’m a computational mechanics researcher at the University of Rey Juan Carlos in Madrid under the supervision of Professor Miguel Otaduy. Currently working on elasticity simulation, my research consists in increasing speed and accuracy through coarsening methods.

I’m looking for a job! I will be applying to both industry and academic positions. I’m particularly interested in roles where I can apply the research I conducted during my PhD. Here’s my Resume.

Research interests: Finite element method, elastic simulation, microstructures, homogenization, coarsening.

Experience

• Research intern at CLO3D • Summer 2023
• Researcher at Multimodal Simulation Laboratory • 2019-Present

Education

• Universidad Rey Juan Carlos : PhD in Computer Graphics • 2019-2024
• Universitat Polytecnica de Catalunya : Master in Numerical Methods for Engineering • 2018-2019
• SeaTech Engineering School : Master in Computational Mechanics • 2016-2019
• University of Toulon : BSc in Sciences for Engineers • 2014-2016

Skills

• Coding [Matlab, Python(JAX), C++ (Eigen)]
• Languages [French native, English fluent certified, Spanish fluent certified]
• Software [Blender, Gimp, Abaqus]
• Research [Physically Based Animation, Microstructures, Homogenization]

Publications

Chan-Lock, A., Otaduy, M
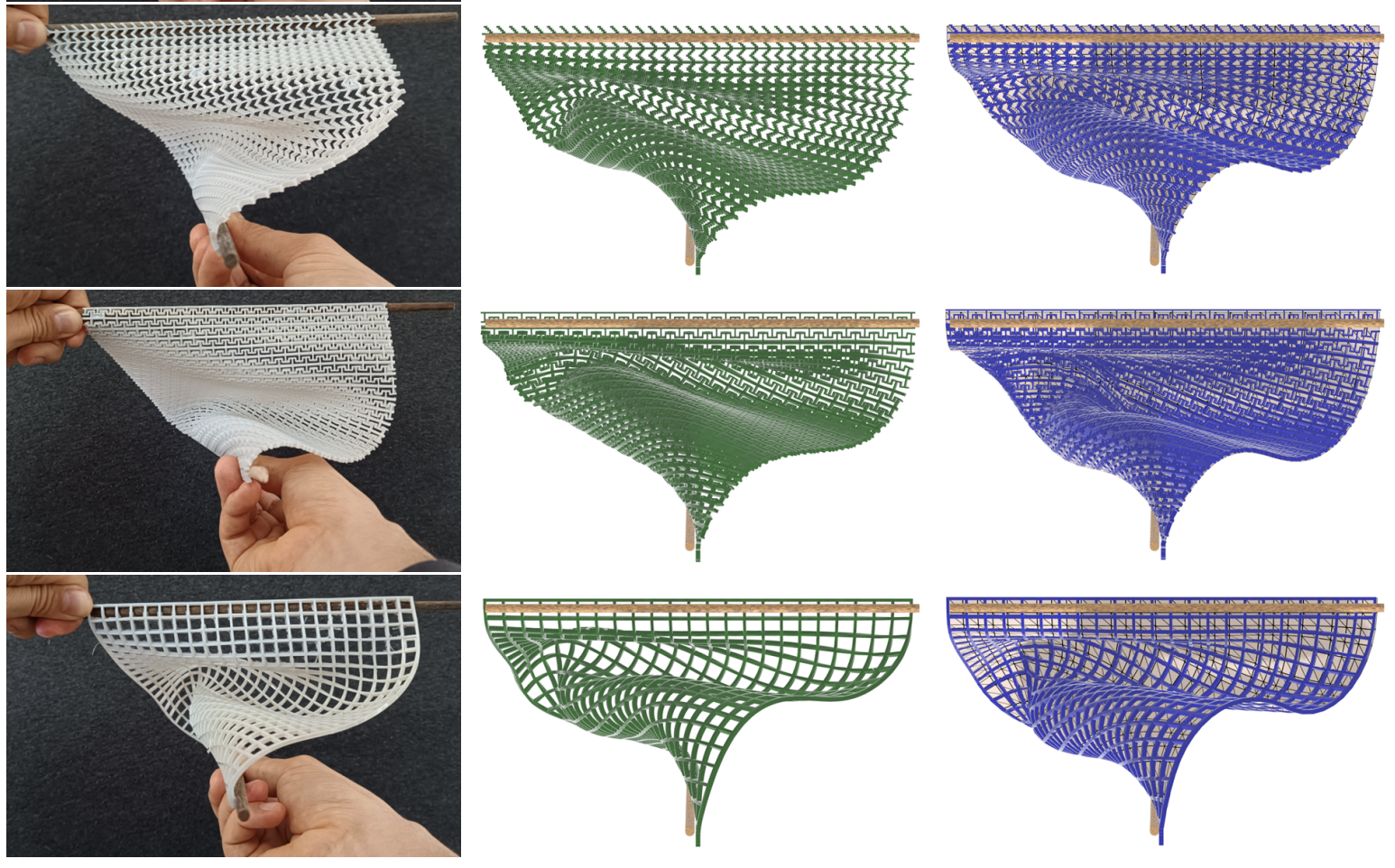
Polar Interpolants for Thin-Shell Microstructure Homogenization

In SIGGRAPH ASIA (Conference), 2024.

This paper presents a new approach to material homogenization for thin-shell microstructures, overcoming key limitations of prior methods. Existing techniques either neglect visual impact (energy-based fitting), lack conservatism (stress-based fitting), or oversimplify the interplay between deformation modes. Our formulation ensures conservative material energy functions, captures high-dimensional interactions between membrane and bending deformations, aligns material domains with training data, and optimizes stress-based parameters for better visual fidelity. Central to our approach is a novel high-order RBF interpolant for polar coordinates, enabling these advancements. The resulting material function and workflow achieve superior quantitative and qualitative fitting of diverse microstructure behaviors.

CitePaper - Video - Code

Chan-Lock, A., Perez, J. Otaduy, M
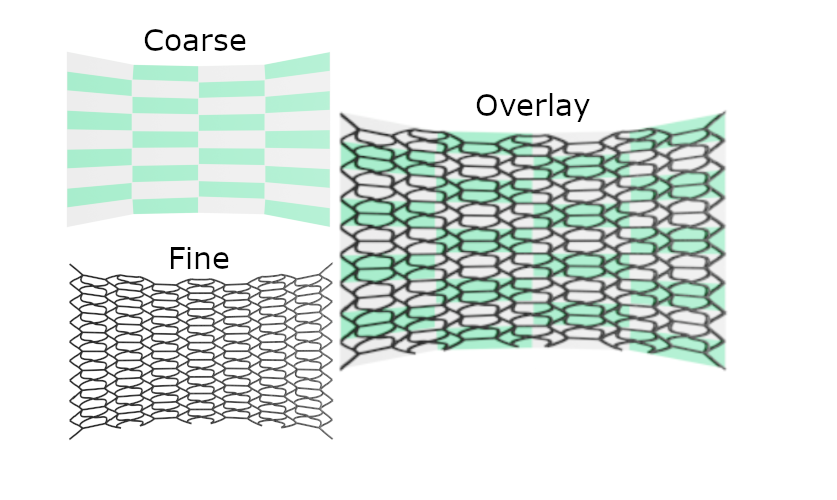
High-Order Elasticity Interpolants for Microstructure Simulation

In Computer Graphics Forum (Proc. SCA), 2022.

We propose a novel formulation of elastic materials based on high-order interpolants, which fits accurately complex elastic behaviors, but remains conservative. The proposed high-order interpolants can be regarded as a high-dimensional extension of radial basis functions, and they allow the interpolation of derivatives of elastic energy, in particular stress and stiffness. Given the proposed parameterization of elasticity models, we devise an algorithm to find optimal model parameters based on training data. We have tested our methodology for the homogenization of 2D microstructures, and we show that it succeeds to match complex behaviors with high accuracy.

CiteMain URLPaper - Video - Code

@article{article,
 author = {Chan-Lock, Antoine  and Otaduy, Miguel A.},
 year = {2024},
 title = {Polar Interpolants for Thin-Shell Microstructure Homogenization},
 journal = {SIGGRAPH Asia Conference Papers (SA Conference Papers ’24)},
 }

@article{article,
 author = {Chan-Lock, Antoine and Perez, Jesus and Otaduy, Miguel A.},
 year = {2022},
 title = {High-Order Elasticity Interpolants for Microstructure Simulation},
 volume = {41},
 number = {8}, journal = {Computer Graphics Forum (Proc. SCA)},
 }